Mastering the concept of "nearest thousandth" is essential for anyone dealing with numbers, whether in academics, finance, or everyday life. This mathematical principle allows us to round numbers to their closest thousandth place, simplifying complex figures while maintaining precision. In this article, we will explore the significance of rounding to the nearest thousandth, how it works, and why it matters in various contexts.

Whether you're a student learning basic math or a professional requiring accurate numerical computations, understanding this concept can significantly enhance your ability to work with numbers efficiently. By grasping how to round numbers correctly, you ensure consistency and accuracy in your calculations.

Our guide will provide a detailed explanation of the nearest thousandth concept, step-by-step methods for rounding numbers, practical examples, and real-world applications. We aim to make this complex topic accessible and easy to understand for readers of all levels.

What is Nearest Thousandth?
How to Round to Nearest Thousandth?
Importance of Nearest Thousandth
Practical Examples of Nearest Thousandth
Common Mistakes in Rounding
Applications of Nearest Thousandth
Sub-Applications in Real-Life Scenarios
Mathematical Significance
Sub-Topics in Mathematical Context
Conclusion

What is Nearest Thousandth?

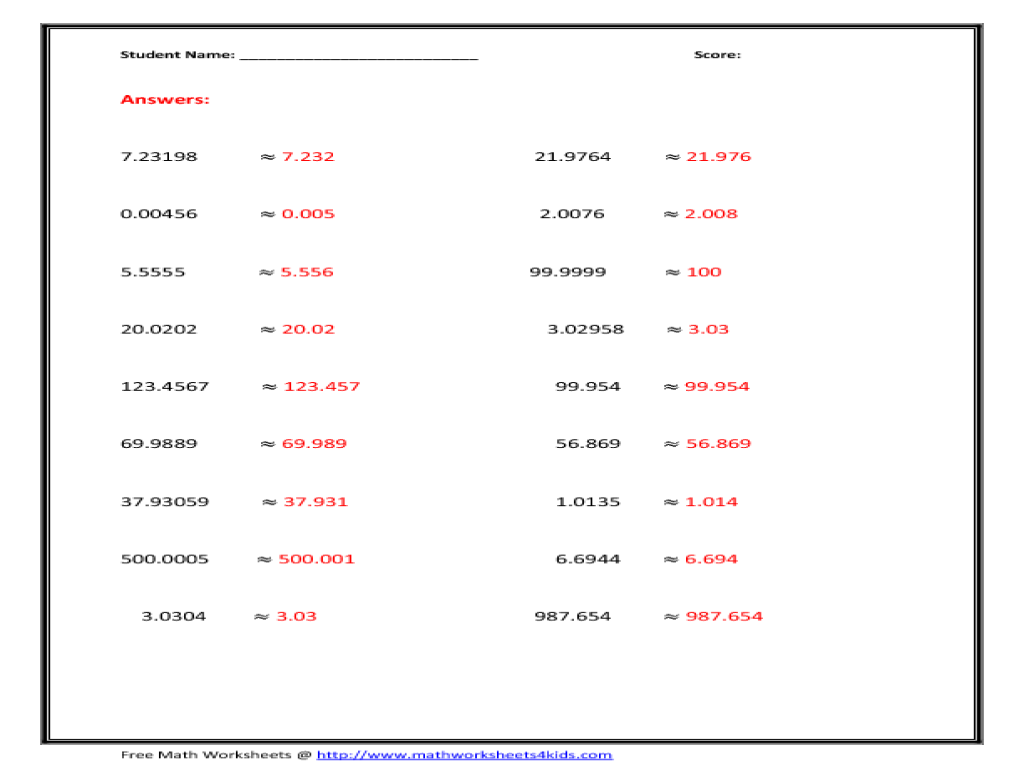
The term "nearest thousandth" refers to the process of rounding a number to the third decimal place. In mathematics, this concept is crucial for simplifying numbers while preserving their approximate value. The thousandth place is the third digit to the right of the decimal point.

For instance, the number 3.14159 rounded to the nearest thousandth becomes 3.142. This rounding technique is widely used in various fields, such as engineering, finance, and scientific research, to ensure that calculations are manageable yet precise.

Understanding the thousandth place and its role in rounding is foundational for mastering more advanced mathematical concepts. It forms the basis of numerical approximation and data representation.

How to Round to Nearest Thousandth?

Step-by-Step Guide

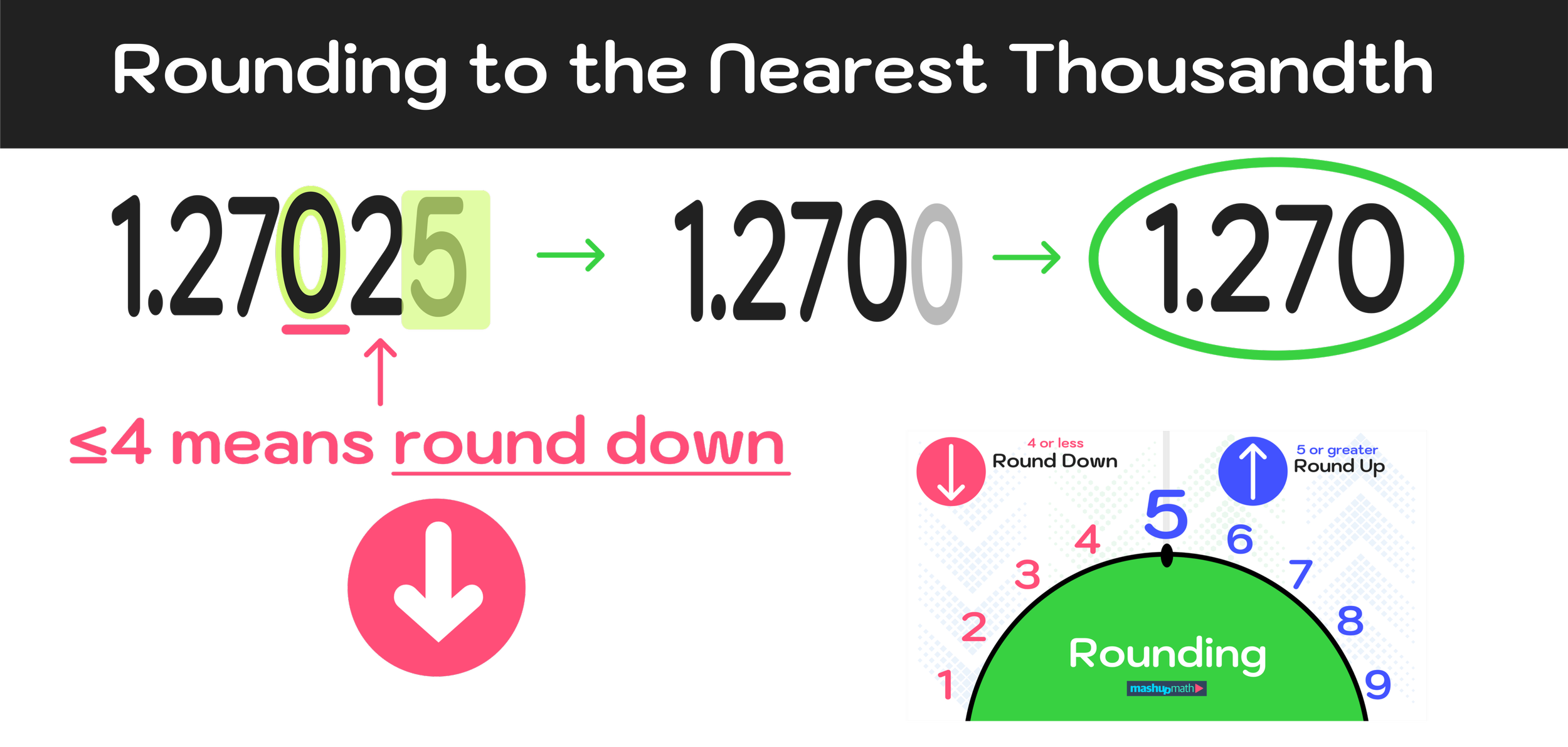
Rounding to the nearest thousandth involves a straightforward process. Follow these steps:

Identify the thousandth place (third digit to the right of the decimal point).
Look at the digit immediately to the right of the thousandth place.
If this digit is 5 or greater, round up the thousandth place by one.
If the digit is less than 5, keep the thousandth place unchanged.

For example, rounding 7.8964 to the nearest thousandth:

The thousandth place is 6.
The digit to the right is 4, which is less than 5.
Therefore, the rounded number is 7.896.

This method ensures consistent and accurate rounding across all numerical contexts.

Importance of Nearest Thousandth

Why Does It Matter?

Rounding to the nearest thousandth is vital for several reasons:

Simplicity: It simplifies complex numbers, making them easier to read and work with.
Accuracy: It maintains a high level of precision while reducing unnecessary decimal places.
Efficiency: It streamlines calculations, saving time and effort in data analysis.

In scientific research, for instance, rounding to the nearest thousandth ensures that results are presented in a standardized format, facilitating comparison and interpretation.

Practical Examples of Nearest Thousandth

Real-World Scenarios

Here are some practical examples of rounding to the nearest thousandth:

Finance: When calculating interest rates or currency conversions, rounding ensures that figures are manageable and consistent.
Engineering: Engineers often round measurements to the nearest thousandth to simplify complex calculations without sacrificing accuracy.
Healthcare: In medical dosages, rounding to the nearest thousandth ensures precise medication administration.

Each of these examples demonstrates the practical importance of this mathematical concept in everyday life.

Common Mistakes in Rounding

Avoiding Errors

When rounding to the nearest thousandth, common mistakes include:

Incorrectly identifying the thousandth place.
Failing to consider the digit to the right of the thousandth place.
Over-rounding or under-rounding numbers.

To avoid these errors, always double-check your calculations and ensure that you follow the correct rounding rules.

Applications of Nearest Thousandth

Where Is It Used?

The concept of rounding to the nearest thousandth has numerous applications across different industries:

Science: Used in data analysis and experimental results.
Technology: Applied in software algorithms for numerical computations.
Education: Taught as a fundamental mathematical skill in schools.

These applications highlight the versatility and importance of this concept in various professional and academic settings.

Sub-Applications in Real-Life Scenarios

Specific Examples

Here are some specific examples of sub-applications:

In weather forecasting, rounding temperature readings to the nearest thousandth ensures precise data representation.
In manufacturing, rounding measurements to the nearest thousandth guarantees product quality and consistency.
In sports analytics, rounding performance metrics to the nearest thousandth provides accurate insights into athlete performance.

These examples demonstrate how rounding to the nearest thousandth enhances accuracy and reliability in various fields.

Mathematical Significance

Why Is It Important in Math?

From a mathematical perspective, rounding to the nearest thousandth is significant for several reasons:

It simplifies complex numbers for easier computation.
It maintains consistency in numerical representation.
It aligns with standard mathematical conventions.

Mathematicians rely on this concept to ensure that calculations are both precise and manageable, especially in advanced mathematical models and equations.

Sub-Topics in Mathematical Context

Advanced Concepts

Some advanced mathematical topics related to rounding include:

Significant Figures: Understanding how rounding affects significant figures in calculations.
Error Propagation: Analyzing how rounding errors impact overall results in complex computations.
Numerical Analysis: Applying rounding principles in algorithms and computational methods.

These sub-topics delve deeper into the mathematical implications of rounding, offering insights into its broader significance.

Conclusion

Rounding to the nearest thousandth is a fundamental mathematical concept with wide-ranging applications in various fields. By mastering this skill, you can simplify complex numbers, maintain accuracy in calculations, and enhance your overall numerical proficiency.

We encourage readers to practice rounding techniques and explore real-world applications to deepen their understanding. Feel free to leave comments or questions below, and don't hesitate to share this article with others who may benefit from it. For further reading, explore our other articles on mathematics and related topics.